September 2020: examples only?

Learning maths by gawping at Google Image search results

Shortish one this time. I'm kind of on a roll with physics at the moment and mostly want to keep my attention on that. None of it is really ready for even newsletter ramblings at the moment, but after finishing the Bell's theorem post I got started on the followup, 'Worse than quantum mechanics', which is going to talk about hypothetical systems that violate the Bell inequality more strongly than anything in QM. (The Popescu-Rohrlich box is the most well-known of these. I can't quickly find a good introductory reference to link to... google it yourself if you're interested, I guess?) Hopefully this will also tie back nicely to my negative probability posts from last year. This one requires some calculations and general fiddling around, so it'll take a while, but I'm enjoying the process.

I'm also highly aware that there's a whole string of shitshows coming up, with second wave covid, the US election and I don't even know what Brexit nonsense (keep forgetting it's even happening what with everything else). So that's probably going to destroy my focus within a month or two. I want to plough on while I still can.

My original plan was to continue with Berlin’s The Roots of Romanticism, and I still want to do that, but it's a bit time-consuming so I'll push it forward. Instead I decided to raid my list of Notebook Blog Month ideas from June to find an easyish topic. So I'm going to talk about...

Examples only

One of my favourite blog posts ever is Timothy Gowers's Examples First. Well, there's actually two blog posts, and the second one is also very good. (In particular it has about the most extraordinary comment section I've ever seen. I once compiled a list of highlights from it, because it just goes on and on with the sort of background cultural insights that nobody ever tells you as a confused undergrad maths student. Golden age of the blogosphere, I suppose.)

As you might expect, it's about putting examples first:

Open a textbook about some general concept in mathematics — Banach algebras, say — and the chances are very high that it will start with a formal definition of Banach algebras and only then give you a few examples. I myself became consciously aware of the principle as a result of editing the Princeton Companion to Mathematics: over and over again I found that I could make an article clearer by putting the authors’ well-chosen examples earlier in their discussion.

Why should it be better to do it that way round? Well, if a general definition is at all complex, then you will have quite a lot to hold in your head. This can be difficult, but it is much easier if the various aspects of the definition can be related to an example with which you are familiar. Then the words of the definition cease to be free-floating, so to speak, and instead become labels that you can attach to bits of your mental picture of the example.

By now the alert reader will have noticed that I have not practised what I have preached. So let’s forget all about the discussion so far and start again, this time doing things properly…

Anyway, I want to talk about a method I've been using quite successfully recently that I've been thinking of as 'examples only'. 'Image search first' might be another good name. It's not for deep understanding, it's mainly for when you want to apply something quickly in a kind of pragmatic way. Maybe you learn the details later once you've seen it in use, maybe you just don't ever care.

So, here's a couple of examples. I tweeted about this first one before. I've been reading a lot of Samson Abramsky's papers recently, and he uses cohomology as a tool fairly often, and I know basically nothing about that (though I'm starting to piece together the prerequisites). So a lot of the time I don't know what's going on. Anyway at some point, either in the papers or as part of my general background googling around to understand them the words 'Čech complex' came up. Sounds pretty intimidating, right? And the text part of the Wikipedia description *is* quite intimidating:

But there’s also an image:

Maybe it starts to make sense if you peer at that for a while? A Google image search is even better, because you can see multiple versions and spot the pattern:

So, really, it's not too bad. Draw a point for every circle. If they intersect draw a line between them. If three intersect draw a filled in triangle. If four intersect draw a tetrahedron... so you've got a simplicial complex (that's another phrase where an image search gets you a lot of the way). I already knew why simplicial complexes were important, so that really was all I needed to know about Čech complexes at that point.

(And yes, I know it’s a lot more abstract and general than just ‘circles’, bla bla bla metric spaces etc. This was enough for me to get the point though.)

Here's another example. I've never really learned any graph theory, but a little while back I wanted to know about the graph Laplacian for some reason (I think I was wondering about whether it would make sense to use in some toy model). And I really appreciated this Wikipedia example:

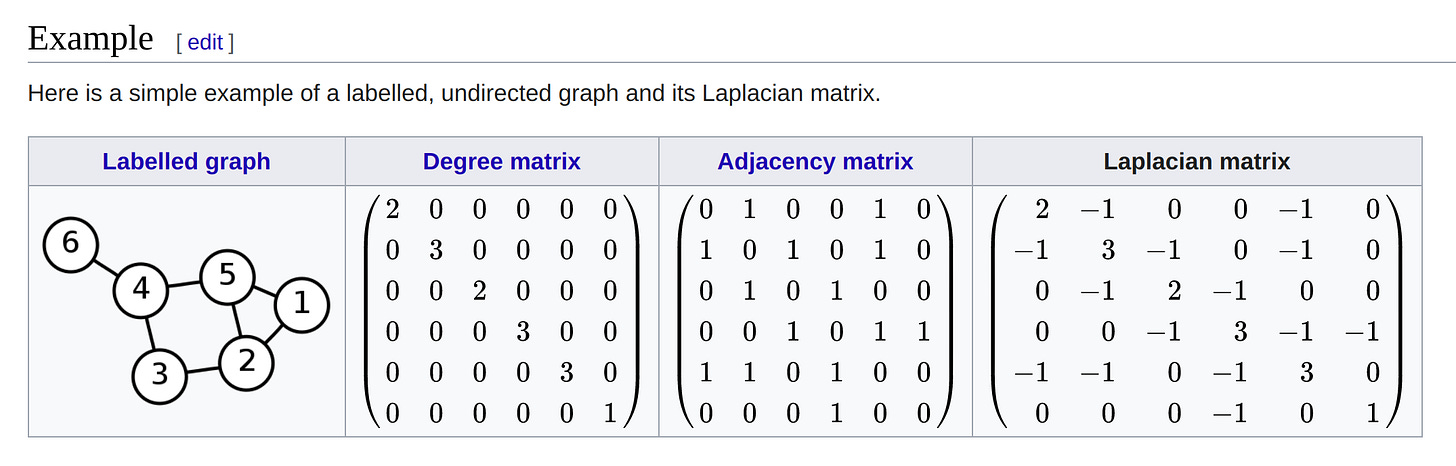
It tells you how to calculate the thing without reading a word of the article... and as a bonus tells you what the degree matrix and adjacency matrix are too. You just have to spend a bit of time staring at the graph and figuring out what properties of it are captured by the matrices. I wish there was one of these right at the top of pretty much any Wikipedia maths article.

Of course, this 'examples only' method works best for things that are highly geometric. And it only gives you a very superficial understanding. But I actually like to start on examples when I only have a very superficial understanding, because I won't care at all about the theory until I've seen it in use. So it works well for me.

It's also more fun. These sort of examples have a puzzle quality where you have to do a bit of work to figure out the connection, and then get a satisfying insight pop when you see it. I'd actually buy a big coffee table book of these, where you get an example, or several, and have to work out what they're examples of. Or a big interactive website would also be good.

On the subject of interactive websites, I was reading Distill's review article earlier this month and my favourite one was Omar Shehata's Unravelling the JPEG, from the Parametric Press. This had a similar example-based setup. There’s a JPEG of a kitten, and next to it is a byte editor:

You can fiddle around changing the numbers, and start to get an idea of what they do:

This worked well for me because after messing around for a bit I was immersed in the problem and actually cared about how it worked. So I really enjoyed following through the various layers of compression and encoding in the later sections, despite having no prior interest in the topic. I find that I glance off a lot of ‘interactive’ articles because there isn’t really much of an effort to draw you in… there are some interactive features, yes, you can move a slider or click a map, but you’re investigating a question that’s just been dumped in front of you and that you have’t built up any particular interest in. Still feels very precanned and uninspiring. This was much better.

Next month

I'm going to keep going with physics. One problem with my current topic is that it has a lot of actual calculations, more than the previous post which was already pushing it for a blog post. So I think I'm going to revive that site I made for physics notes and never use and post all the boring details there first. Not sure anyone will ever read them, but they'll be useful to me as a reference, and I can link to them in the post, sort of like a paper appendix. So I'll focus on that next month, and I'm not sure whether I'll get to the blog post as well.

And I'm going to plug my ears and shout LA LA LA CAN'T HEAR YOU for as long as I can in response to all the upcoming shitshows in the news. It won't last, but I can try.

Cheers,

Lucy